Recently, a colleague and I were working on a show in a highly reverberant space. Although we only had a few vocal microphones on stage, the room was an acoustic challenge, with a really nasty 80 Hz mode that rang like a bell for more than 2 seconds.

I asked my colleague if he was driving the system’s subwoofer via an aux bus, and he replied that he wasn’t, but that he was using high-pass filtering on the mic inputs. This prompted a discussion as to whether the two methods are equally effective at cleaning up the low end of a mix.

First, let’s define the issue.

A quick look with a spectrum analyzer will confirm that there is often a significant amount of sub-100 Hz signal content picked up by vocal mics on stage. This low-frequency energy consists of vocal pops and plosives, wind rumble, stage noise, traffic noise, handling noise, HVAC noise, and other annoyances.

In all but a few specific situations, this LF energy is unwanted clutter, usually manifesting as an annoying rumble from the subwoofers. The “booms” associated with vocal pops are typically the worst offenders – on plosive peaks, the energy between 60 and 80 Hz can actually exceed the level of the desired in-band vocal content if left uncorrected.

Obviously the optimal solution is to use the right microphone, and several models exist that are designed specifically for these types of problems. However, as is often the case, this was a “this is what we have to work with” situation.

Available Options

Aside from mic choice, there are two main defensive strategies: using high-pass filters on all inputs that don’t have useful LF information, and feeding the subwoofers from a post-fader auxiliary send (aux-fed subs or AFS) containing only the channels that do have useful LF information.

As a starting point, let’s look at a system that’s configured as left/right crossed over to the sub and tuned to a flat response.

It would be tempting to think that a 100 Hz high-pass (or “low-cut”) filter effectively removes all energy below 100 Hz, but this is not the case. High-pass filters (HPF) are, by definition, -3 dB at the chosen corner frequency (except for Linkwitz-Riley crossover filters, which are -6 dB since they’re made from two cascaded Butterworth filters).

From there, the steepness of the rolloff depends on the order of the filter, with each filter pole supplying 6 dB per octave rolloff. A second-order filter has a slope of 12 dB per octave, third order a slope of 18 dB per octave, and so on. The HPF does a good job reducing energy at frequencies far below the cutoff, but the critical 60 to 80 Hz region is a different story: it’s often too close to the filter’s cutoff frequency to have an effective amount of attenuation.

To quantify this, I measured a few console high-pass filters (Figure 1). The horizontal yellow line highlights -3 dB to easily compare the corner frequency of each filter. The yellow-shaded vertical rectangle indicates the critical 63 to 80 Hz “pop region” that I’m looking to target with the HPF.

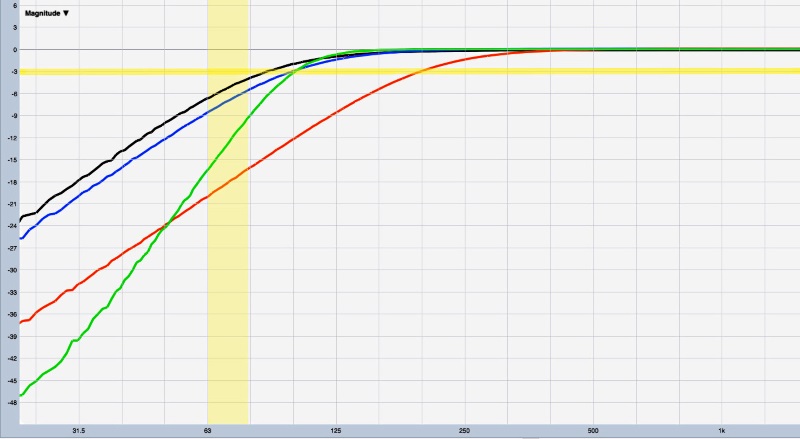
First up is the black trace, which shows the response of the fixed-frequency HPF on a small-format analog mixer. According to the label on the console itself, it’s an 80 Hz filter, but the analyzer reveals the actual -3 dB point around 87 Hz. (This brings up the question of whether the console’s stated EQ parameter values can be trusted. For a look at this, see Bend Me Shape Me.)

Only 3 to 6 dB of attenuation in the pop region doesn’t go too far towards a solution. (Note that the LF vocal pop issue can be made worse with systems tuned with a “sub bump” or with the subs gained up higher than the full-range mains. If an HPF is supplying 6 dB of attenuation at a given frequency but the subs are turned up by the same amount, you’re effectively back where you started.)

The blue trace shows an HPF response from a digital console from the same manufacturer. We can see that the slope is the identical second order (12 dB per octave) response, and since we have the flexibility of a sweepable filter, I’ve tuned it to 100 Hz, which nets about 3 dB more rejection in the pop zone without crowding the vocal signal. Being 9 dB down at 60 Hz isn’t as much as I’d like, but it’s an improvement.

As an experiment, I raised the corner frequency of the filter until the vocal pops through the system sounded adequately controlled, and ended up with a corner frequency of 200 Hz (Figure 1, orange curve). The second order slope means that the very effective 15 dB+ of rejection in the “pop zone” comes at the price of intrusion into the vocal range, which is undesirable as my goal is just to control the plosives, not alter the tone of the vocalist’s performance.

Informed Decisions

This was a nice little reality check for me, as I’ve clocked a lot of hours on this model of console and have always felt like I had to run the HPF up higher than I would on other desks to tame the plosives. This is confirmed by the fact that the filter is a shallower slope than that of the filters on other consoles I work with. Of course, we want to use our ears, not our eyes, when making EQ decisions, so there’s nothing inherently wrong with seeing a high corner frequency onscreen, but I do prefer a steeper slope in general for this task.

The green trace shows another popular digital console’s HPF, also set to 100 Hz. The steeper slope here (fourth order, 24 dB per octave) means more attenuation where it counts, without interfering with the in-band signal.This is certainly not a one-size-fits-all solution – sometimes a steeper HPF slope is a problem-solver, and in other applications it’s not wanted or needed.

(It warrants a mention here that although the phase shift produced by such a steep slope is inaudible in itself, the group delay issues may be problematic when considered in the context of the entire signal chain.)

Some consoles offer two slope options for their channel strip HPF, but barring that, what else can we do if a steeper slope is desired? At the event that prompted this discussion, my colleague was running the channel’s HPF all the way up to 200 Hz and not getting the rejection he needed, so used one of the console’s graphic EQs inserted on the channel (Figure 2) to achieve significantly more attenuation.

The red trace in Figure 3 shows the resulting curve as compared to the previous HPF settings of 100 and 200 Hz.

One the one hand, it solved the problem and gave him the results he needed. On the other hand, you’re not alone if you think this is an inefficient solution to the issue. It might do the job with a single-input show, but it’s not going to scale up for larger productions.

This is the real appeal of aux-fed subs. No filtering required – the LF energy from the mics simply can’t get to the sub, period, unless I decide to route it there. The constant low rumble is effectively banished from the system – Dave Rat once likened the improvement to “turning off an air conditioner in a room.”

The technique seems to have gained some bad PR, probably due to people who use it as an opportunity to run their subs a thousand dB hotter than the rest of the system.

At one outdoor event, I mentioned to a guest engineer that we had the option of patching his desk in with separate drive for the subs if he’d like, and he said “No, I like to hear the whole show.”

For me, aux-fed subs is about cleaner, not louder. I personally choose to run my subs via an aux whenever possible based on the requirements of the show. That being said, it’s not a foolproof solution, and there are still a few things to keep in mind.

The Right Balance

For starters, having the subs on a separate drive can make the system harder to measure and optimize, because what the measurement mic hears won’t match the reference signal sent to the analyzer. I tune the system as a standard crossed-over LR system and then simply route a different drive signal to the sub line once optimization is complete, without changing filters or gains.

Another point: for the same reason that high-pass filters are not a perfect solution, even with aux-fed subs the main system will still be putting out some LF energy below the crossover point (which on larger-format PA systems can be 80 Hz or even lower) and so a channel HPF is still likely to be helpful.

In the end, it’s not an either-or solution. I’ve been able to achieve great results using a combination of both approaches, and understanding the concepts behind both allow me to make the best decision for the show.